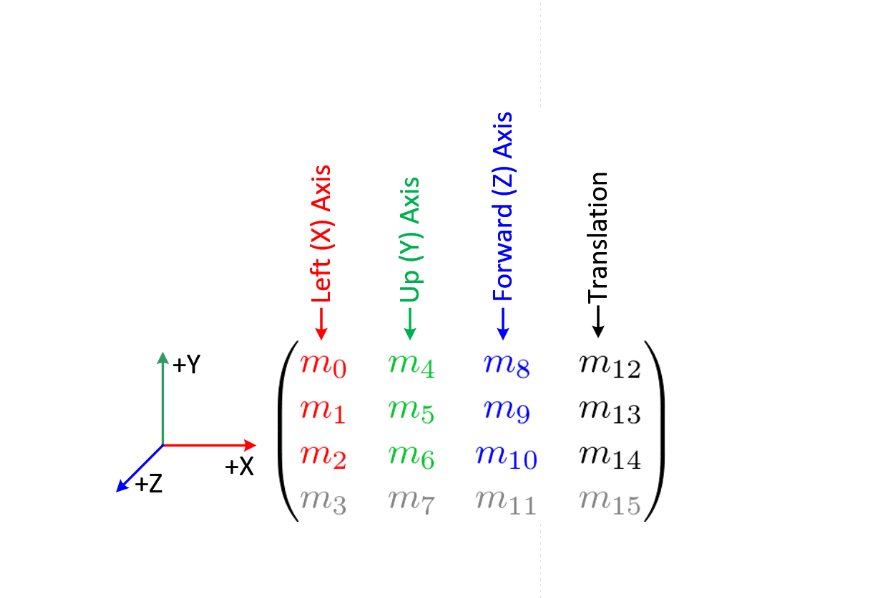
👗 🏣 🛏️ ¿Por qué estamos transformando vectores 3D con matrices 4x4? 🧛🏾 👩🏽‍🤝‍👩🏼 🤸🏻

¿Por qué no una matriz de 3x3? ¿Por qué todo está organizado en una matriz de 4x4 exactamente así? ¿Por qué la última línea está llena de ceros y uno al final? Hice estas preguntas el día anterior, decidí investigar la pregunta y contar lo que descubrí.

En el artículo, solo nos interesarán las transformaciones afines y, en particular, la rotación, el escalado y el movimiento, que se utilizan activamente en la programación gráfica y el desarrollo de juegos en general.

: . , , , (A⋅x). , , , (+b).

T, x

$T (\ vec {x}) = A \ vec {x} + \ vec {b}$

, b x . x x', :

$\ begin {array} {ll} A \ rightarrow [a] \ rightarrow a, \\ \ vec {b} \ rightarrow (b) \ rightarrow b, \\ \ vec {x} \ rightarrow x, \\ T (x ) \ rightarrow x '\ end {matriz}$

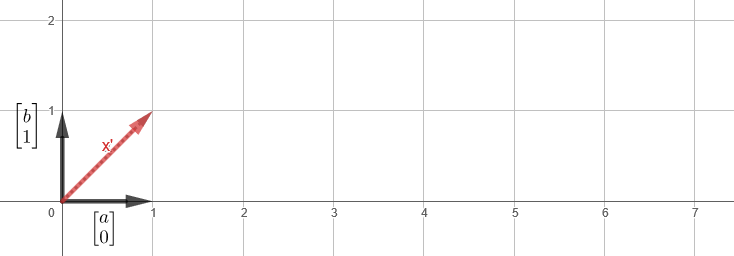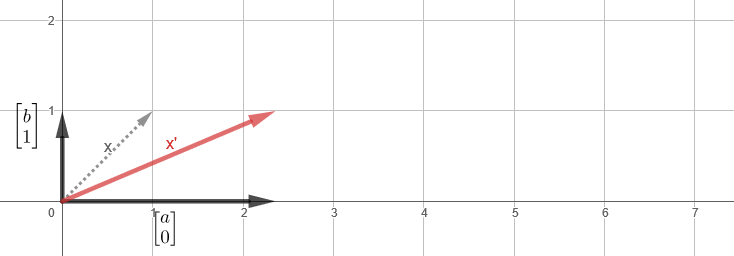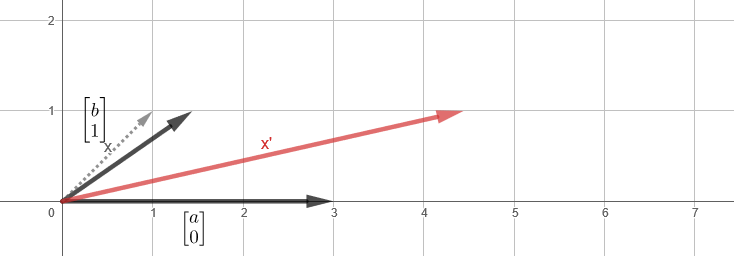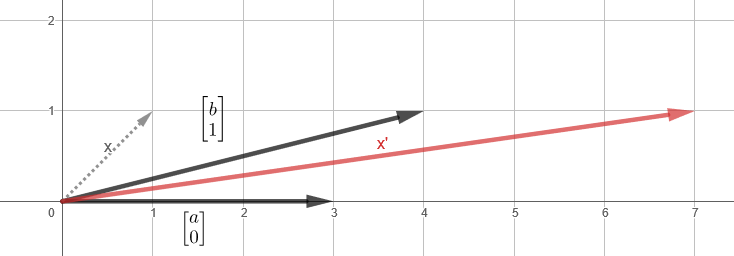
x ( ), b ( ).

, , . M, :

x' = 3x + 4 (3x +4 ) .

$\ begin {matriz} {ll} Mx = 3x + 4 \\ M = (3x + 4) / x \\ M = 3 + (\ frac {4} {x}) \ end {matriz}$

, ( 3x [3]), (x+4) , M x.

:

+4 +4y, y, x ,

$x '= 3x + 4y \\ y' = \ _x + \ _y$

2x2, x' = 3x+4 x, . . , .

$\ begin {bmatrix} x '\\ y' \ end {bmatrix} = \ begin {bmatrix} 3 & 4 \\ \ _ & \ _ \ end {bmatrix} \ begin {bmatrix} x \\ y \ end {bmatrix }$

2x2 , , - y, +4y , +4, x :

$\ begin {bmatrix} x '\\ y' \ end {bmatrix} = \ begin {bmatrix} 3 & 4 \\ \ _ & \ _ \ end {bmatrix} \ begin {bmatrix} x \\ 1 \ end {bmatrix } \ rightarrow \ begin {array} {l} x '= 3 \ cdot x + 4 \ cdot 1 \\ y' = \ _ \ cdot x + \ _ \ cdot 1 \ end {array}$

, , , , 3x+4 x' - y' y' ,